Black And Scholes Calculator Excel

The Black-Scholes model is a widely used financial model that estimates the value of a call option or a put option. The model was developed in 1973 by Fischer Black and Myron Scholes, and it has become a cornerstone of modern finance. In this article, we will discuss the importance of the Black-Scholes model and how to use an Excel calculator to estimate the value of options.

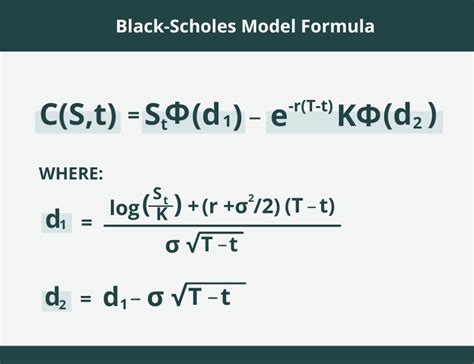
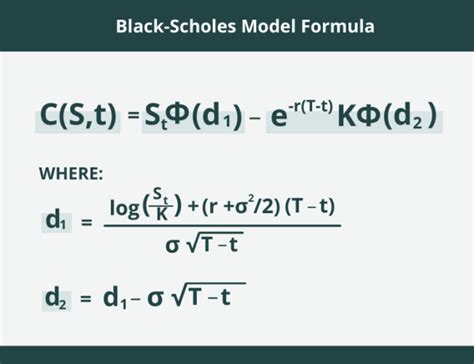
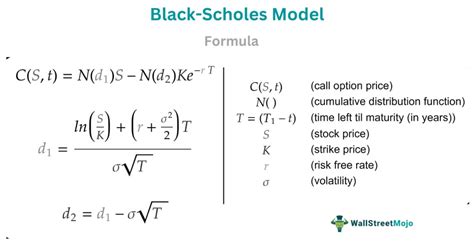
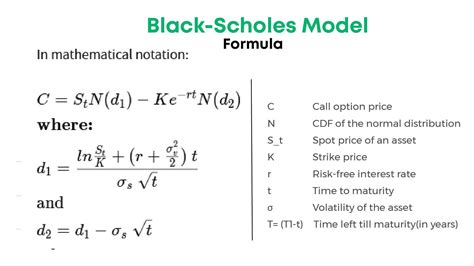
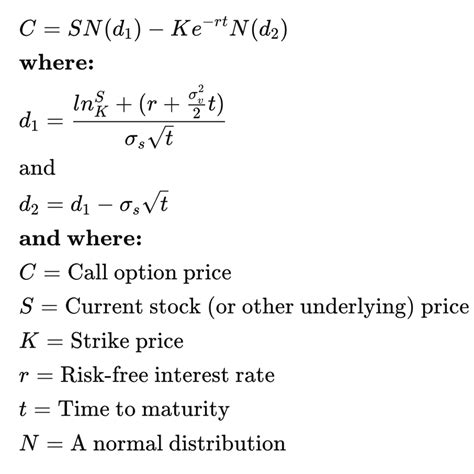
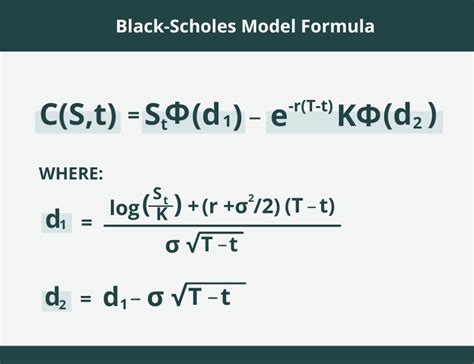
The Black-Scholes model is a complex mathematical formula that takes into account several factors, including the current stock price, the strike price of the option, the time to expiration, the risk-free interest rate, and the volatility of the underlying asset. The model is based on the assumption that the price of the underlying asset follows a geometric Brownian motion, and it uses a risk-neutral valuation approach to estimate the value of the option.

One of the key advantages of the Black-Scholes model is that it provides a simple and intuitive way to estimate the value of options. The model can be used to estimate the value of call options and put options, and it can also be used to estimate the value of more complex options, such as binary options and barrier options.

To use the Black-Scholes model, you need to input several parameters, including the current stock price, the strike price of the option, the time to expiration, the risk-free interest rate, and the volatility of the underlying asset. The model then uses these parameters to estimate the value of the option.

Introduction to Black-Scholes Calculator Excel

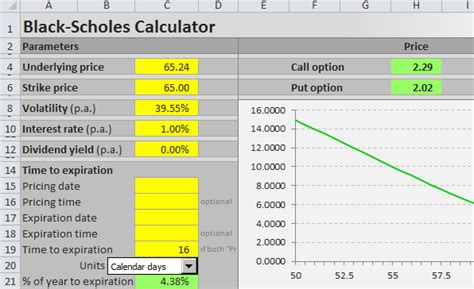
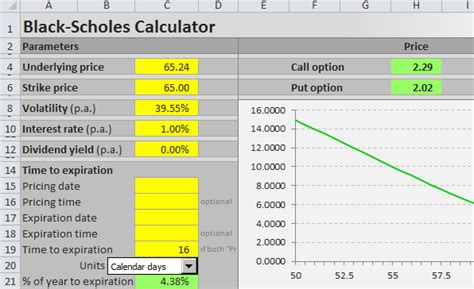
A Black-Scholes calculator Excel is a spreadsheet that uses the Black-Scholes model to estimate the value of options. The calculator typically includes several input fields, where you can enter the parameters required by the Black-Scholes model. The calculator then uses these parameters to estimate the value of the option and displays the result in a output field.

Using a Black-Scholes calculator Excel can be a convenient way to estimate the value of options, especially if you need to estimate the value of multiple options with different parameters. The calculator can save you time and effort, and it can also help you to avoid errors that can occur when using the Black-Scholes model manually.

How to Use a Black-Scholes Calculator Excel

To use a Black-Scholes calculator Excel, you need to follow these steps:

1. Open the calculator spreadsheet and enter the required parameters, including the current stock price, the strike price of the option, the time to expiration, the risk-free interest rate, and the volatility of the underlying asset.
2. Select the type of option you want to estimate the value for, either a call option or a put option.
3. Click the calculate button to estimate the value of the option.
4. The calculator will display the estimated value of the option in a output field.

Benefits of Using a Black-Scholes Calculator Excel

There are several benefits of using a Black-Scholes calculator Excel, including:

• Convenience: The calculator can save you time and effort, especially if you need to estimate the value of multiple options with different parameters.
• Accuracy: The calculator can help you to avoid errors that can occur when using the Black-Scholes model manually.
• Flexibility: The calculator can be used to estimate the value of different types of options, including call options and put options.
• Customization: The calculator can be customized to meet your specific needs, including the ability to input your own parameters and formulas.

Limitations of the Black-Scholes Model

While the Black-Scholes model is a widely used and well-established financial model, it has several limitations, including:

• Assumptions: The model is based on several assumptions, including the assumption that the price of the underlying asset follows a geometric Brownian motion.
• Simplifications: The model simplifies several aspects of the option pricing process, including the assumption that the risk-free interest rate is constant.
• Volatility: The model assumes that the volatility of the underlying asset is constant, which may not always be the case.

Common Applications of the Black-Scholes Model

The Black-Scholes model has several common applications, including:

• Option pricing: The model is widely used to estimate the value of options, including call options and put options.
• Risk management: The model can be used to estimate the risk of an option position and to determine the optimal hedge ratio.
• Portfolio optimization: The model can be used to optimize a portfolio of options and to determine the optimal allocation of assets.

Real-World Examples of the Black-Scholes Model

The Black-Scholes model has several real-world examples, including:

• Option trading: The model is widely used by option traders to estimate the value of options and to determine the optimal trading strategy.
• Risk management: The model is used by companies to estimate the risk of their option positions and to determine the optimal hedge ratio.
• Portfolio optimization: The model is used by portfolio managers to optimize their portfolios of options and to determine the optimal allocation of assets.

Black Scholes Model Image Gallery

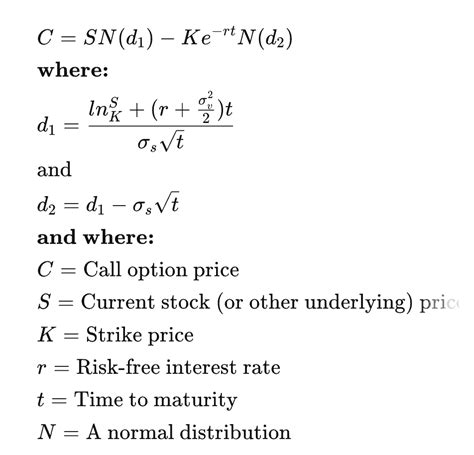
What is the Black-Scholes model?

+

The Black-Scholes model is a financial model that estimates the value of a call option or a put option. The model was developed in 1973 by Fischer Black and Myron Scholes, and it has become a cornerstone of modern finance.

How does the Black-Scholes model work?

+

The Black-Scholes model uses a complex mathematical formula to estimate the value of an option. The formula takes into account several factors, including the current stock price, the strike price of the option, the time to expiration, the risk-free interest rate, and the volatility of the underlying asset.

What are the limitations of the Black-Scholes model?

+

The Black-Scholes model has several limitations, including the assumption that the price of the underlying asset follows a geometric Brownian motion, and the assumption that the volatility of the underlying asset is constant. The model also simplifies several aspects of the option pricing process, including the assumption that the risk-free interest rate is constant.

In conclusion, the Black-Scholes model is a widely used financial model that estimates the value of a call option or a put option. The model is based on a complex mathematical formula that takes into account several factors, including the current stock price, the strike price of the option, the time to expiration, the risk-free interest rate, and the volatility of the underlying asset. While the model has several limitations, it remains a cornerstone of modern finance and is widely used by option traders, companies, and portfolio managers. We invite you to share your thoughts and experiences with the Black-Scholes model, and to explore the various resources and tools available to help you master this powerful financial model.