Intro
The concept of distance, speed, and time is fundamental in understanding how objects move and how we can calculate various aspects of their motion. This relationship is often represented by the formula: Distance = Speed × Time. Understanding this formula and being able to apply it to different scenarios is crucial in physics, engineering, and everyday life. In this article, we'll delve into the world of distance, speed, and time, exploring their definitions, the relationship between them, and how to apply this knowledge in practical problems.
Distance, speed, and time are interrelated and can be used to solve a wide range of problems. For instance, if you know how far you need to travel (distance) and how fast you can go (speed), you can calculate how long your journey will take (time). Conversely, if you know the speed at which you're traveling and the time you've been moving, you can determine how far you've gone. This basic principle is used in everything from planning road trips to calculating the orbits of satellites.
To better grasp these concepts, let's define them:
- Distance refers to how far apart objects are. In the context of motion, it's the total length of the path covered by an object.
- Speed is a measure of how fast an object is moving. It's defined as the distance traveled per unit time.
- Time is what we use to measure the duration of an event or the interval between events.
Understanding the Distance Speed Time Formula

The formula Distance = Speed × Time (D = ST) is the cornerstone of understanding the relationship between these three variables. This formula can be rearranged to solve for any of the variables if the other two are known. For example, to find speed, you would use the formula Speed = Distance / Time (S = D/T), and to find time, you would use Time = Distance / Speed (T = D/S).
Practical Applications
The distance-speed-time relationship has numerous practical applications:
- Travel Planning: When planning a trip, knowing the distance to your destination and the speed at which you'll be traveling allows you to calculate the time it will take to get there.
- Physics and Engineering: Understanding how objects move is crucial for designing everything from roller coasters to space missions.
- Sports: Athletes and coaches use this relationship to optimize performance, whether it's improving running times or calculating the trajectory of a thrown ball.
Solving Distance Speed Time Problems

To solve problems involving distance, speed, and time, follow these steps:
- Identify the Knowns and Unknowns: Determine what information you have and what you're trying to find.
- Choose the Right Formula: Use the formula that isolates the variable you're solving for.
- Plug in the Values: Insert the known values into the formula.
- Solve for the Unknown: Perform the necessary calculations to find the answer.
Examples and Exercises
Here are a few examples to practice:
- If a car travels at an average speed of 60 km/h for 2 hours, how far does it go?
- A bike rider covers a distance of 20 miles in 2 hours. What is the rider's average speed?
- A plane needs to travel 3000 miles in 5 hours. What speed must it maintain?
Tips for Mastering Distance Speed Time Calculations

To become proficient in solving distance-speed-time problems:
- Practice regularly with a variety of problems.
- Ensure you understand the units of measurement and convert them if necessary.
- Double-check your calculations for accuracy.
Real-World Applications and Career Implications

Understanding distance, speed, and time is essential for careers in:
- Aerospace Engineering: Designing spacecraft and missions requires precise calculations of distance, speed, and time.
- Transportation Planning: Urban planners and transportation engineers use these principles to design efficient road networks and public transportation systems.
- Physics Research: Scientists studying motion and forces need a deep understanding of how distance, speed, and time relate.
Distance Speed Time Worksheet
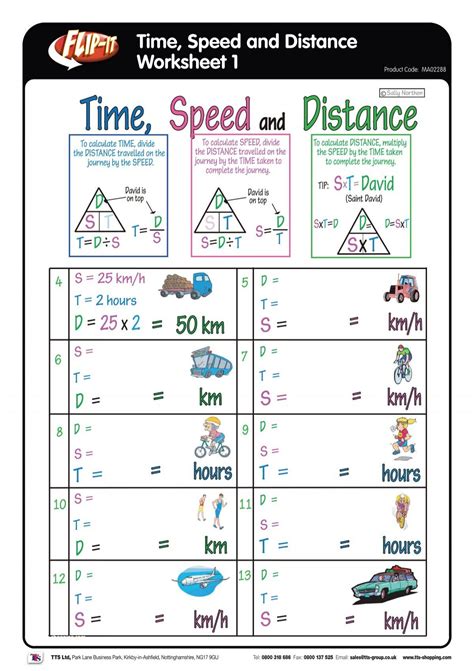
A worksheet typically includes a variety of problems that test your ability to apply the distance-speed-time formula in different scenarios. These can range from simple calculations to more complex problems involving multiple steps or converting between units.
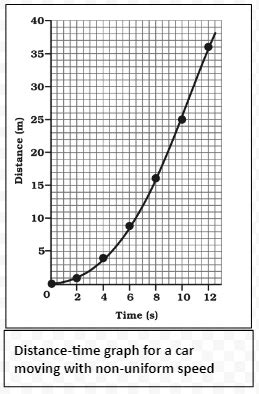
Gallery of Distance Speed Time Problems
Distance Speed Time Image Gallery
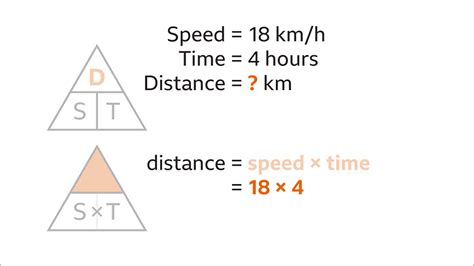
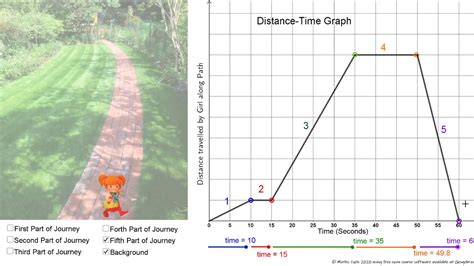



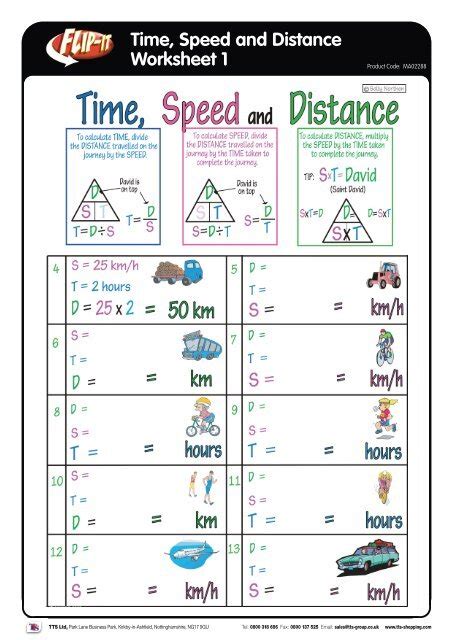
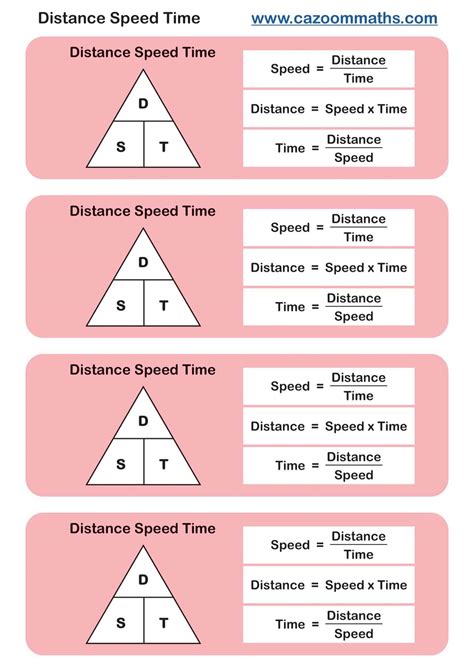
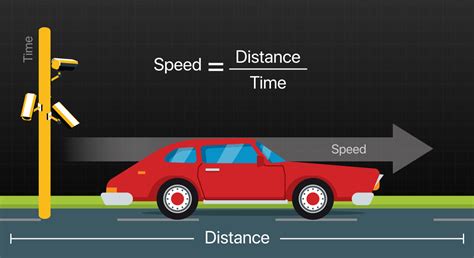
What is the formula for calculating distance using speed and time?
+The formula to calculate distance is Distance = Speed × Time (D = ST).
How do you calculate speed if you know the distance and time?
+To find speed, use the formula Speed = Distance / Time (S = D/T).
What are some real-world applications of understanding distance, speed, and time?
+Understanding distance, speed, and time is crucial in fields like aerospace engineering, transportation planning, and physics research.
In conclusion, mastering the concepts of distance, speed, and time is essential for a wide range of applications, from everyday travel planning to complex scientific and engineering challenges. By understanding the formula that relates these three variables and practicing with various problems, individuals can develop a strong foundation in physics and improve their problem-solving skills. Whether you're a student looking to excel in science and math or a professional seeking to apply these principles in your work, the distance-speed-time relationship is a fundamental tool that can help you achieve your goals. We invite you to share your thoughts on the importance of distance, speed, and time in the comments below and to explore more resources on this topic to deepen your understanding.