Intro
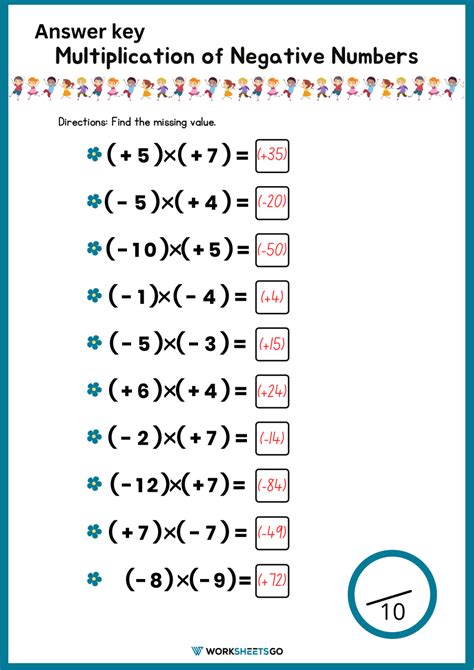
Multiplying negative numbers is a fundamental concept in mathematics that can seem daunting at first, but with practice and understanding, it becomes straightforward. The rule for multiplying negative numbers is simple: when you multiply two negative numbers, the result is positive, and when you multiply a negative number by a positive number, the result is negative. This concept is crucial in various mathematical operations and real-world applications. Let's dive into the world of negative numbers and explore how to multiply them with ease.
To start, it's essential to understand the basics of negative numbers. Negative numbers are numbers that are less than zero. They can be represented on the number line to the left of zero. When you multiply a negative number by another negative number, you are essentially performing an operation that yields a positive result. For example, -3 multiplied by -4 equals 12, because the two negative signs cancel each other out, resulting in a positive product.
On the other hand, when you multiply a negative number by a positive number, the result is always negative. This is because the negative sign takes precedence, indicating that the result is less than zero. For instance, -5 multiplied by 6 equals -30, demonstrating how the negative sign influences the outcome of the multiplication.
Understanding these rules is crucial for solving multiplication problems involving negative numbers. It's also important to remember that the rules apply regardless of the order in which you multiply the numbers. The commutative property of multiplication states that the order of the factors does not change the product. Therefore, whether you start with a negative number and then multiply by a positive number, or vice versa, the result will be the same.
Benefits of Practicing Multiplication of Negative Numbers
Practicing the multiplication of negative numbers has several benefits. It helps reinforce the understanding of negative numbers and how they interact with positive numbers in mathematical operations. Additionally, it prepares students for more advanced mathematical concepts, such as algebra and geometry, where negative numbers play a significant role. Regular practice also improves mental math skills, enabling individuals to perform calculations more quickly and accurately.
Real-World Applications
The concept of multiplying negative numbers is not limited to theoretical mathematics; it has numerous real-world applications. In physics, for example, negative numbers are used to represent directions. When calculating the result of forces acting in opposite directions, multiplying negative numbers becomes essential. Similarly, in economics, negative numbers can represent debts or losses, and understanding how to multiply them is crucial for financial calculations and projections.Steps for Multiplying Negative Numbers

To multiply negative numbers, follow these simple steps:
- Identify the Signs: Determine the signs of the numbers you are multiplying. Are they both negative, or is one negative and the other positive?
- Apply the Rule: If both numbers are negative, the result will be positive. If one is negative and the other is positive, the result will be negative.
- Multiply the Numbers: Ignore the signs for a moment and multiply the numbers as you normally would.
- Apply the Sign: Based on the rule, apply the appropriate sign to your product.
Examples and Practice
Let's consider a few examples to solidify our understanding: - Multiplying two negative numbers: -2 * -5 = 10 (Both numbers are negative, so the result is positive.) - Multiplying a negative and a positive number: -3 * 4 = -12 (One number is negative and the other is positive, so the result is negative.)For more practice, consider the following exercises:
- -1 * -1 =?
- -2 * 3 =?
- 4 * -5 =?
Answers:
- 1
- -6
- -20
Common Mistakes and How to Avoid Them

One of the most common mistakes when multiplying negative numbers is forgetting to apply the correct sign to the product. To avoid this, always remember the rule: two negatives make a positive, and a negative and a positive make a negative. Another mistake is not following the order of operations, especially in expressions involving multiple operations. Always follow the PEMDAS rule (Parentheses, Exponents, Multiplication and Division from left to right, Addition and Subtraction from left to right) to ensure that operations are performed in the correct order.
Tips for Better Understanding
To better understand and work with negative numbers, consider the following tips: - **Use the Number Line**: Visualizing numbers on a number line can help understand how negative numbers work and how they interact with positive numbers. - **Practice Regularly**: The more you practice, the more comfortable you will become with multiplying negative numbers. - **Apply to Real-Life Situations**: Try to find examples of negative numbers and their multiplication in real-life scenarios to make the concept more relatable and interesting.Conclusion and Next Steps

In conclusion, multiplying negative numbers is a fundamental mathematical concept that, with practice and patience, becomes easy to grasp. By understanding the simple rules and applying them consistently, you can master the multiplication of negative numbers. Remember, practice is key, so keep challenging yourself with different examples and exercises. As you become more proficient, you'll find that working with negative numbers becomes second nature, opening the door to more complex and fascinating areas of mathematics.
Final Thoughts
The journey to mastering negative numbers is ongoing, and there's always more to learn. Whether you're a student looking to improve your grades or an individual seeking to enhance your mathematical skills, remember that every step forward is a step in the right direction. Keep exploring, stay curious, and most importantly, enjoy the process of learning and discovery.Multiplication of Negative Numbers Image Gallery






What is the rule for multiplying two negative numbers?
+The rule states that when you multiply two negative numbers, the result is always positive.
How do you multiply a negative number by a positive number?
+When multiplying a negative number by a positive number, the result is always negative.
Why is practicing the multiplication of negative numbers important?
+Practicing the multiplication of negative numbers helps reinforce the understanding of negative numbers and prepares individuals for more advanced mathematical concepts.
We hope this comprehensive guide to multiplying negative numbers has been helpful and informative. Whether you're a student, teacher, or simply someone looking to improve your mathematical skills, remember that practice and patience are key. If you have any questions or would like to share your experiences with multiplying negative numbers, please don't hesitate to comment below. Your feedback and insights are invaluable in helping others understand and master this important mathematical concept.